Journal of Industrial and Systems Engineering

Journal of Industrial and Systems Engineering

Vol. 1, No. 1, pp 70-80

Spring 2007



A Project Scheduling Method Based on Fuzzy Theory



Ahmad Soltani1,* , Rasoul Haji2



1 Sharif University of Technology and Engineering Research Institute, Ministry of



Agricultural Jahad, P. O. Box: 13445-754, Tehran, Iran

(Asoltani@Eri.ac.ir)

2Department of Industrial Engineering, Sharif University of Technology, P. O Box:



11365-9414, Tehran, Iran

(haji@sharif.edu)



ABSTRACT



In this paper a new method based on fuzzy theory is developed to solve the project scheduling

problem under fuzzy environment. Assuming that the duration of activities are trapezoidal

fuzzy numbers (TFN), in this method we compute several project characteristics such as earliest

times, latest times, and, slack times in term of TFN. In this method, we introduce a new

approach which we call modified backward pass (MBP). This approach, based on a linear

programming (LP) problem, removes negative and infeasible solutions which can be generated

by other methods in the backward pass calculation. We drive the general form of the optimal

solution of the LP problem which enables practitioners to obtain the optimal solution by a

simple recursive relation without solving any LP problem. Through a numerical example,

calculation steps in this method and the results are illustrated.



Keywords: Project scheduling, Fuzzy theory, Modified backward pass (MBP), Trapezoidal

fuzzy number (TFN), Linear programming (LP)



1. INTRODUCTION:



Scheduling is deemed to be one of the most fundamental and essential bases of the project

management science. There are several methods for project scheduling such as CPM, PERT and

GERT. Since too many drawbacks are involved in methods estimating the duration of activities,

these methods lack the capability of modeling practical projects. In order to solve these problems, a

number of techniques like fuzzy logic, genetic algorithm (GA) and artificial neural network can be

considered.



A fundamental approach to solve these problems is applying fuzzy sets. Introducing the fuzzy set

theory by Zadeh in 1965 opened promising new horizons to different scientific areas such as project

scheduling. Fuzzy theory, with presuming imprecision in decision parameters and utilizing mental

models of experts is an approach to adapt scheduling models into reality. To this end, several

methods have been developed during the last three decades. The first method called FPERT, was

proposed by Chanas and Kamburowski (1981). They presented the project completion time in the

form of a fuzzy set in the time space. Gazdik (1983) developed a fuzzy network of an a priori

unknown project to estimate the activity duration, and used fuzzy algebraic operators to calculate

the duration of the project and its critical path. This work is called FNET. An extension of FNET



*

Corresponding Author


----------------------- Page 2-----------------------

A Project Scheduling Method… 71



was proposed by Nusution (1994) and Lorterapong and Moselhi (1996). Following on this,

McCahon (1993), Chang et al. (1995), and, Lin and Yao (2003) presented three methodologies to

calculate the fuzzy completion project time. Other researchers such as Kuchta (2001), Yao and Lin

(2000), Chanas and Zielinski (2001), and, Oliveros and Robinson (2005), using fuzzy numbers,

presented other methods to obtain fuzzy critical paths and critical activities and activity delay.

Previous work on network scheduling using fuzzy theory provides methods for scheduling projects.



These methods, however, do not support backward pass calculations in direct manner similar to that

used in the forward pass. This is mainly due to the fact that fuzzy subtraction is not proportionate to

the inverse of fuzzy addition. Therefore, these methods are incapable to calculate project

characteristics such as the latest times and slack times.



In this paper a new method is introduced for project scheduling in fuzzy environment. This method

is developed based on a number of assumptions and definitions in the fuzzy set and project

scheduling. In the fuzzy project network considered in this paper, we assume that the duration of

activities are trapezoidal fuzzy numbers (TFN). The project characteristics such as fuzzy earliest

times and fuzzy project completion time are calculated as TFN by forward pass. As mentioned

above, backward pass in fuzzy environment fails to compute the fuzzy latest times and fuzzy slack

times. Therefore, for computation of these parameters we propose a new approach which we call

modified backward pass (MBP).



This approach is based on the project scheduling fundamental concepts and linear programming. In

MBP, using the project scheduling concepts, fuzzy latest times and fuzzy slack times relations are

transformed to linear programming (LP) problem. After that, we drive the general form of the

optimal solution of the LP problem which enables practitioners to obtain the optimal solution by a

simple recursive relation without solving any LP problem.



The advantage of MBP approach in comparison with the previous approaches is that it does not use

the fuzzy subtraction operator in its relations. Due to, these modifications, the inherent defects

discussed before in the fuzzy environment will remove. Therefore, the obtained fuzzy latest times

and fuzzy slack times in the MBP approach are correct and calculated as TFNs, as well. Finally,

through a numerical example, calculation steps in this approach and result are illustrated.



2. DEFINITIONS AND ASSUMPTIONS



In this section, some basic notions of the area of fuzzy theory that have been defined by Kaufmann

and Gupta (1985) and Zimermann (1996), are introduced. Then, project network is defined as a

directed and acyclic graph in fuzzy environment.



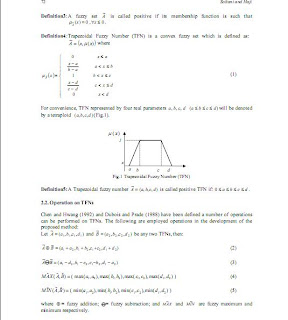
2.1. Definitions